Fan Efficiency Calculation: Formula & Equation

Table of Contents

Together with the advent of equipment efficiency legislation and the growing awareness of ownership costs, fan efficiency has become a critically important selection factor. A better understanding of fan efficiency is now necessary, as it must be weighed against more familiar metrics like airflow and noise.

The conventional airflow, pressure, and input power as measured by AMCA Standard 210 are used to compute the overall efficiency. The effectiveness of the motor or the drive (belt drive) is not considered. The air power divided by the fan input power is how fan efficiency is calculated. Fans are available from a variety of Suppliers and Companies, as well as various manufacturers and distributors, and there are several Fans for Sale on Linquip.

There is a complete list of Fan Services on the Linquip website that covers all factory operations. Linquip providers can assist you with this. Please contact Linquip’s Fan Experts to learn more about how to connect with a diverse group of service providers who consistently deliver high-quality items.

This article discusses fan efficiency in some detail and provides a PDF for more information.

Fundamental Concepts

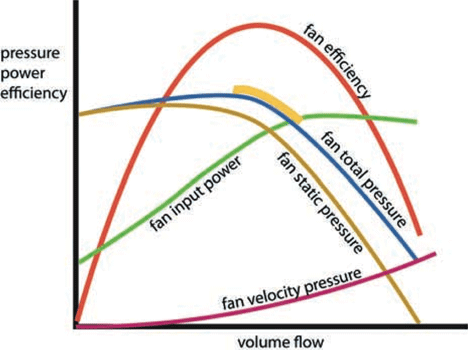
Positive static pressure is created as air passes through a system by means of fans. All other components in the airflow path produce negative static pressure by resisting the movement of air. In order to compensate for the negative static pressure caused by system obstructions, different fan types generate different airflow values.

14 HVAC Fan Reference airassurance.com fan efficiency — HVAC Fan (Reference **airassurance.com**)

Manufacturers generally suggest static efficiency as the efficiency value, whereas total efficiency includes outlet velocity. Based on total pressure, the total efficiency of a fan is calculated. However, calculating static efficiency only considers static pressure. In total pressure, the static pressure and velocity pressure are added together. In general, outlet velocity pressure does not influence a fan’s capability to dissipate heat energy from a system, so it is not considered in the efficiency calculation.

Dynamic Pressure

A velocity pressure (or dynamic pressure) indicated by q in incompressible fluid dynamics is defined as:

$q=\frac{1}{2}\rho u^2$

In the equation above, q is measured in pascals (or kgm⁻¹s⁻²). ρ is fluid density (in kg/m³) and u is the velocity of flow (in m/s).

Static Pressure

Pressure is an essential concept in fluid science. There can be a pressure measurement anywhere within a fluid body, regardless of its motion. A variety of methods can be used to measure pressure, including an aneroid, Bourdon tube, mercury column, and others.

Based on Bernoulli’s equation, the concept of total pressure and dynamic pressure is important in understanding the behavior of fluids. Neither of these two pressures can be measured using an aneroid, Bourdon tube, or mercury column. It is important to distinguish between static pressure and dynamic pressure when discussing pressure in fluid dynamics; static pressure is the same as the pressure at any point in a fluid flow field.

Among the fundamental equations of fluid dynamics is Bernoulli’s equation. For most fluid flow applications, elevation changes are negligible. In this simplified form, Bernoulli’s equation for incompressible flows becomes:

$p+\frac{1}{2}\rho u^2=p_0$

p is the static pressure, and p₀ represents total pressure. The value of total pressure is constant along any streamline. This pressure is also called stagnation pressure. Another expression for the above equation is:

$static\ pressure\ +\ dynamic\ pressure\ =\ total\ pressure$

The figure below shows the different fan performance curves in terms of airflow.

How to Calculate Fan Efficiency

The efficiency of a fan as an energy converter is determined by the ratio of its output to the input power:

$\eta =\frac{P_{out}}{P_{in}}$

Input power delivered to a fan is:

$P_{in}=V\times I$

V and I are the electrical voltage (in Volts) and amperage (in Amps). P is measured in Watts. In Metric units, fan output power (P_out) is calculated as:

$P_{out}=pQ$

In this equation, p is the air pressure in pascals and Q is the airflow in m³/s.

Aerodynamic loading has a dramatic effect on fan efficiency. A fan operating in the free air condition (without any backflow pressure) will have zero pressure, and so zero airpower, and therefore, zero efficiencies. A fan in a full shut-off state (no flow) also produces no airpower and has zero efficiencies. Typically, an axial fan’s efficiency is at its highest at around 1/3rd the maximum pressure.

In general, the greater the blade diameter and the faster the speed, the more efficient the fan will be. In recent years, fan manufacturers have taken steps to develop fans with higher peak efficiencies compared to older designs.

Fan Efficiency Grade (FEG)

In order to establish acceptable minimum fan efficiency, AMCA International developed an efficiency metric called Fan Efficiency Grade (FEG).

FEG is a simple method of calculating the aerodynamic quality of the fan and is based on its maximum total efficiency. Calculating the total efficiency requires using airflow, pressure, and input power. This factor disregards the efficiency of the drive (belt drive) and motor. You can calculate both the static and total efficiency of a fan from its performance data as follows:

$Static\ Efficiency=\frac{CFM\times p}{6343\times BHP}$

$Total\ Efficiency=\frac{CFM\times p_0}{6343\times BHP}$

CFM represents fan flow rate (it can also be denoted by Q) in ft/m³, p is static pressure in inches of water (in. wg), and p₀ is total pressure (in. wg). Also, BHP is Fan power input measured in hp.

High-Efficiency Fans

Modern high-end servers generate a great deal of heat, which requires more power to cool. Due to this, more electricity will have to be allocated to the cooling system’s components. A high-end rack system may require more than 25% of the total power budget for cooling fans.

When high-efficiency fans are used, they have a cascade effect on the design of the system. It may be possible to downsize power supplies in order to save weight and space and lower the power distribution network for fans.

A high-efficiency fan will reduce the cost of ownership in the long run. A large data center can have up to ten thousand servers with between ten and fifty fans in each of them. The efficiency of each installed fan can be improved by several percentage points, resulting in energy savings of many thousands of dollars each year.

Fans with high efficiency can be more expensive than older types, which may act as a deterrent. A purchaser or engineer should be cognizant of the wider implications of using the latest fan designs. The lower power requirements can lead to system-level savings, while substantial savings for consumers can be realized.

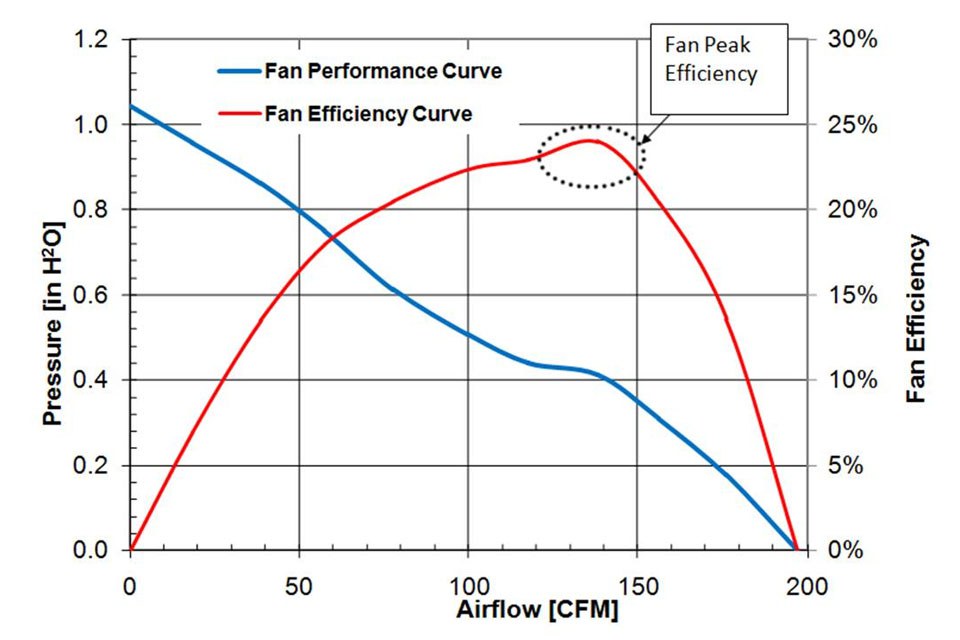
The following figure shows both total and static fan efficiency plotted with the fan curve. Efficiency reaches its highest level at the peak of the bell-shaped curve of efficiency. The FEG value is derived from this peak efficiency.

On the curve, peak efficiency occurs at only one point, and all others are lower. According to the efficiency curves, each fan has a wide range of efficiency depending on airflow and pressure. A fan with a maximum efficiency of 70%, for example, can easily be chosen to operate at 50% efficiency.

Another aspect is that the fan size determines its efficiency. The smaller the fan, the less efficient it is. On larger fans, the smallest dimensions – such as material thicknesses and clearances between parts – can be held tightly in proportion to the other dimensions, but not so for smaller fans. AMCA FEG curves have been established so that fans of a given model that have similar geometric characteristics will have approximately the same grade.

The importance of fan efficiency in the reduction of energy consumption has been discussed in the following PDF.

The Role of Fan Efficiency in Achieving Energy Reduction Goals

Drawbacks of FEG

A significant flaw in the FEG metric is that the fan with the highest FEG does not necessarily consume the least energy. For example, the 72-inch fan consumes the least energy (lowest BHP); however, the 48- inch fan possesses a higher total efficiency (66% vs. 60%) and a greater FEG (71 vs. 63).

It means that a fan that is more efficient uses twice as much energy; but how?

First and foremost, the FEG takes into account both fan total efficiency and fan total pressure. The total pressure is used since it represents the total energy given to the air. As a result, the velocity pressure exiting a fan can only be used in ducts, and on non-ducted fans, it is lost. Consequently, the FEG metric is often misconstrued for many types of fans. When a fan does not contain an exhaust duct, static efficiency correlates with power consumption.

Secondly, the FEG value is determined by the fan’s peak efficiency. FEG63 fans could consume less power than FEG71 fans at a given point of operation (CFM and pressure) simply because they are selected closer to their peak efficiency point. There is a greater potential to operate more efficiently with fans with higher peak efficiencies.

Download Fan Efficiency PDF

Download PDF

Buy Equipment or Ask for a Service

By using Linquip RFQ Service, you can expect to receive quotations from various suppliers across multiple industries and regions.

Click Here to Request a Quotation From Suppliers and Service Providers

Read More In Linquip

2 thoughts on “Fan Efficiency Calculation: Formula & Equation”

Anwar Shiekh
June 12, 2022 at 7:12 AM

“A fan operating in the free air condition (without any backflow pressure) will have zero pressure, and so zero airpower, and therefore, zero efficiency.”

This is missing the kinetic energy; the fan has taken stationary air and given it kinetic energy.

Elfantau
June 17, 2022 at 5:14 AM

Unfortunately, this article is completely useless because no examples are provided to illustrate the equations in a real situation (ex : to make your own fan).